Fundamentals of Radio Astronomy

Joachim Köppen Strasbourg 2010

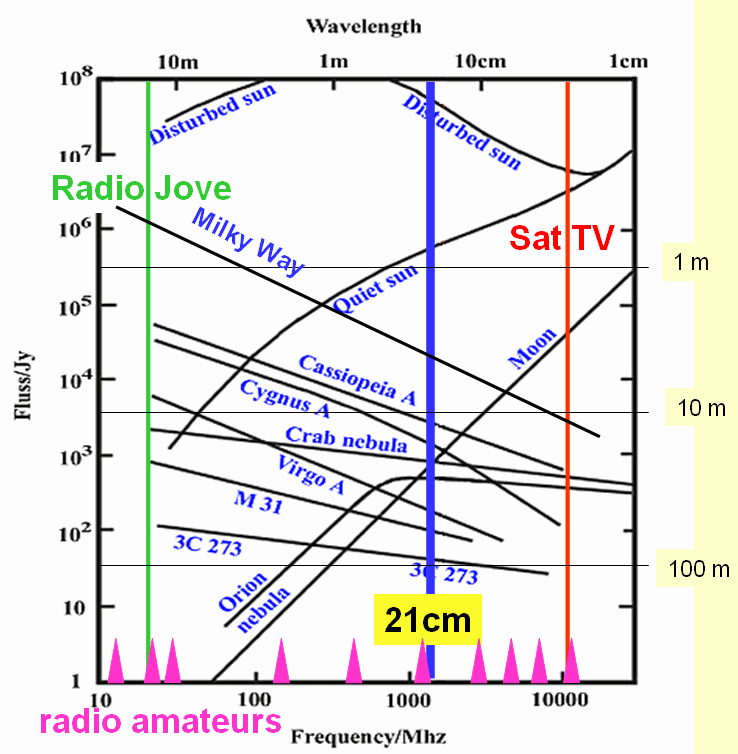
This is the radio spectrum of astronomical sources (from the book "Radioastronomy" by John Kraus, W8JK, interactive version). The green line marks 20 MHz, the frequency of the RadioJove receiver (to capture radio bursts from Jupiter and the Sun; ours was operational between 2003 and 2009, and had to be closed down because the evergrowing electronic pollution had rendered observations useless). The red line marks 12 GHz, where the ESA-Dresden telescope works. The blue line is the frequency of the 21 cm line of atomic hydrogen - at a frequency of 1420.406 MHz - where the ESA-Haystack telescope observes. The vertical axis shows the flux of the sources, that is the power per unit surface area of the telescope. The horizontal lines marked with 1m, 10m, and 100m indicate the sensitivity that can be achieved by radio telescopes of that diameter: they show the fluxes which just equal to the thermal noise produced in the receiver itself (at room temperature).

Introductory material on radio astronomy

There is a wealth of material on various aspects of radio astronomy which is available on the Internet, either by professional institutions or by dedicated amateurs. It is well worth "googling" for a while on the Net to find information suited to one's particular taste and level. Here are a few references that we found quite useful:

• Book on RadioJove telescope by Richard Flagg: this is primarily written for the observation on 20 MHz of Jupiter and the Sun, but this well-written book contains a few chapters which also serve well as an introduction to radio astronomy.
• MIT Haystack Radio Astronomy Tutorial gives a good introduction to the basic concepts.
• Radio Astronomy Course by Dale E.Gary is a graduate lecture course, which is a good introduction to the subject, with good plots and images. Some parts will be too advanced for our purposes. Also, there are a number of interesting links.
• Radio Astronomy on 10 GHz with the ESA-Dresden Radio Telescope contains material about the other telescope in operation at ISU.
• Itty Bitty Radio Telescope is primarily about a very simple approach to 10 GHz radio astronomy, but it also contains some very valuable links for background information.

• SARA is the website of the Society of Amateur Radio Astronomers, and presents a lot of good material and projects.

Some essential definitions and relations

For the interpretation of observations it is necessary to clarify what is it that we measure of the radiation. In particular, we have to distinguish between two quantities, the flux and the intensity. In radio astronomy, we speak of antenna temperature and brightness temperatures:

• the flux (or more accurately: the flux density) F (or often: S) is the radio energy collected by the antenna per second, per unit frequency interval, and per unit surface area. Hence its unit is 1 W/Hz/m² = 1 Ws/m². Note that this quantity is independent of the antenna and the receiver details. It is solely characterised by the source.
• for the small fluxes encountered in radio astronomy it is very convenient to define the unit 1 Jansky = 1 Jy = 1E-26 Ws/m² to measure fluxes. For example, our Sun has at 10 GHz a flux of about 4000000 Jy = 4 MJy, which makes it the brightest natural object in the sky.
• But what we do measure with the receiver is the power (or power density) P per unit frequency, which is related to the flux by P = F * A / 2 where A is the (effective) cross section of the antenna dish. There is also a reduction by a factor 2, because we observe in horizontal or verical polarization only.
• The electronic components in the receiver produce electronic noise because of the thermal motions of the electrons on the components. This noise presents a limit for the minimum signal that can be detected. The thermal noise produced by a resistor of a given absolute temperature T (measured in K for Kelvin) is simply P_noise = k * T with Boltzmann's constant k = 1.38 E-23 Ws/K. Active devices, such as the transistors in the amplifying stages, produce a greater amount of noise!
• For instance, a resistor at room temperature (300 K) provides a power density of about 4 E-21 Ws (or W/Hz), which can be written as 400000 Jy m² = 0.4 MJy m². This means that if our antenna has a cross section of 1 m² (like our dish), it will receive from the Sun about 4 MJy m², one half of which is picked up by the linearly polarized dipole antenna in the telescope's focus: Thus 2 MJy m² reach the receiver, which is only five times as much as the thermal noise that is present in the electronics of the receiver! This means that the signal-to-noise ratio is only 5 ...
• Because of this comparison with the thermal noise it is useful to define the Antenna temperature T_ant by P = k * T_ant as the noise temperature that gives the same amount of power density as the received signal. In our example, the Sun would produce on a 1 m² dish an antenna temperature of T_ant = 1156 K.
• But please keep in mind that while the concept of the antenna temperature is useful for the technical side of reception of signal, it is not related to the properties of the source.

• A somewhat more abstract, but more revealing quantity is the Intensity I of the radiation: this is the power per unit frequency interval passing through a surface of unit area and from (or into) a unit solid angle. The solid angle Ω measures what portion of the entire sky is covered by the source, the entire sky having a solid angle of 4π, being a full sphere. The unit of the intensity is W/Hz/m²/sr (= W/Hz*m²*sr) ... where sr stands for "steradian" and means: per unit solid angle.
• For a small circular source, one can compute the solid angle as Ω = (angular diameter * π/180°)² π/4 which is often given by this approximate formula: Ω = (angular diameter * π/180°)²
• is the source much larger than the antenna beam, the solid angle is that of the antenna: Ω = Ω_ant = λ²/A with the wavelength l. This relation between the solid angle and the effective cross section A of the antenna holds for all antennas.
• Flux and intensity are related by F = I * Ω
• The intensity has a very useful property: along a line of sight in vacuum it does not change, irrespective of the distance from the light source. It changes only, if there are additional light sources or if light is absorbed by intervening material. Thus, the intensity tells us about the nature of the light source.
• The intensity of a body emitting thermal radiation - this is a good approximation for the sun and many other bodies, such as our own bodies! - is reasonably well described by the black-body law (or Planck curve): I = B = 2 h f**3 /c² / (exp(hf/kT) - 1) with Planck's constant h = 6.626 E-34 Ws², the speed of light c = 299790 km/s, and the frequency f.
• For the temperatures of celestial radio sources, the peak of the black-body curve occurs at frequencies much higher than those in the radio range, so that one may use the Rayleigh-Jeans approximation instead: I = B = 2 k T /λ² which means that the intensity is directly proportional to the temperature of a body!
• in radio astronomy it is useful to define the Brightness Temperature I = 2 k T_B /λ² for any source and whatever might be the process producing the radiation. However, for thermal emission this brightness temperature is equal to the temperature of the emitting body, thus it tells about the internal physics of the source. Examples for non-thermal emission are sychrotron emission or maser sources.
• If we measure intensity in Jy/sr, the above formula for the brightness temperature becomes I = 2760 T_B/λ² with the wavelength λ in meter.

| Top of the Page | Back to the MainPage | to my HomePage |

last update: Feb. 2010 J.Köppen