Observations of the Sun

Joachim Köppen Strasbourg 2010

• Basics
• Observational Procedures
• Analysis and Interpretation
• More about our results

Observations of the Sun are quite important, not only because of the Sun, but also to measure important parameters of the telescope itself. At the frequency of 11 GHz the Sun emits a continuum radiation produced by the hot gas which makes up the Sun. However, at radio frequencies the emission does not come from the photosphere that we observe in the visible wavelength range, but from the hotter layers above the photosphere: at 11 GHz we look at the bottom of the Transition Layer between the Chromosphere and the Corona where the temperature starts to rise.

The other aspect is about the telescope: Since the empty sky provides radio wave emission only at negligible intensity, the signal that we measure is essentially the background noise from our receiver. Comparing the solar signal with that of the sky we obtain a measure of the sensitivity of our instrument: The larger the ratio of the solar and sky signals is, more sensitive is the system. We express this more quantitatively as the System Temperature.

The third aspect is also technical: We can determine the width of the antenna pattern, i.e. the Half-Power Beam Width (HPBW) which describes also the angular resolution possible with the antenna. This is done by letting the Sun - which we use as a source with a constant signal - pass through the antenna beam, and measure the time it takes. With the knowledge of the Earth rotation's angular velocity we convert the time interval into an angle, the HPBW.

Observational procedure

Important note: Because the positioning system of this telescope has only a limited accuracy and stability, good observations require the your presence during the observing run, because you have to manually optimize the pointing of the antenna to your source.

After starting up the system, described here, we recommend to follow this sequence:

• from now on: whatever you do, don't forget to keep the Observatory log
• click on goto Calibrator and Start, so that the observation run begins with a flux calibration, and all data are recorded in a file
• Stay at the calibrator a few minutes. The tracing will show some fluctuations on a basically flat curve. After about two minutes you have acquired sufficient data points to permit you to determine a reliable average value
• The choice of the frequency and polarization is not so important, but in later observations you might want to change them from the default values
• click on the Sun now button. The current position of the Sun will be displayed in the text fields. Click on Goto to move the telescope there ...
• However, when the movement stops, you may notice the the current position does not agree with want you had wanted, and the signal maybe also quite low: The positioning system of the telescope is neither sufficiently accurate nor stable to move the antenna to the exact location. By using the direct control keys at the Yeasu controller, and watching the measurements you have to manually find the best position:
  • interrupt your measurements by clicking on Stop: this will cause the receiver to display its measurements more frequently!
  • use the keys on the controller to move the antenna Up, Down, Left, or Right, perhaps with touching the keys only shortly ... until you get a maximum signal
  • but after each move, wait for a couple of measurements: after a move, the antenna wobbles a bit, and furthermore there are always fluctuations of the signal!
  • move about the source until you find the really largest signal. Sometimes, even a slight maneuver or going back a bit may bring the best position ... do not bother about the fact that the displayed position may not have changed!
  • when you are happy with the position, Resume your measurements
• now lean back, wait and watch. wait until the signal goes down, as the Sun moves out of the antenna beam. Wait until the signal becomes constant, so you observe the background from the empty sky ... this takes about 20 minutes. Do wait until the signal is really flat, as you need a good measurement of the background.
• if you had done this, you'll get a half drift scan, so you might not be perfectly sure if you have captured the real peak signal: so you can also try to position the telescope a little bit in advance of the Sun. This is best done around noon, when the Sun moves only horizontally across the sky, so that you do not have to worry about the change in elevation. In this case, simply move manually to the west (Right) a couple of degrees, perhaps as far as you get already the empty sky signal ... and then wait for the signal to rise and to fall again!
• Because of the limited accuracy of the positioning system, you cannot blindly rely on the option Sun +15min! It needs some more tricks to do a good job.
• after you got a good constant signal, goto Calibration and stay there for a few minutes.
• after that, have another go ... and just keep recording the data in the the same file!
• when you are really done with your observations, end the run by clicking Stop+Finish, and shut down the system.
• You find your recorded data in the text file at: C:/JKStuff/, named: UTyear/month/day/time.txt for example: UT1004141630.txt if I observed starting at 16:30 on April 14th 2010 (all times are given in UTC). Copy the file to your USB stick or flash drive to transfer onto your computer.

Analysis

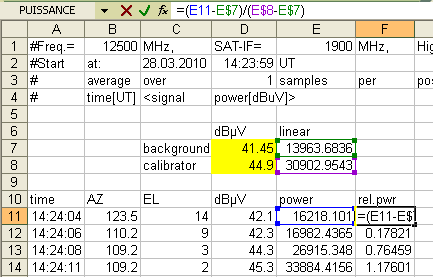
Let's suppose that we use Microsoft Excel to do the interpretation of the data. Then we recommend to follow this sequence:

• To import your text file go to: File -> Import-> Text File -> Select your Text File -> Delimited Text file -> Delimiter is spaces -> Finish
• Each row represents a single measurement taken by the telescope at a certain time instant. The columns from left to right represent: Time, Azimuth, Elevation, Power measured in dBµV.
• We shall need only the times and the power
• First, make a scatter plot of the power as a function of time, which would look like this (which is part of a series of observations):
• Next, insert a couple of rows where you put in some cells the guess parameters for the background and calibrator levels, as seen below: Here I marked with yellow the cells which need my input. Next to them, convert these dBµV values in linear values power_linear = 10^(power_dBµV / 10) to have those values handy
• in the column next to the the one containing the power values, convert all those dBµV values also in linear units.
• in another column, you convert these linear power values into the background-subtracted and calibrated relative powers, using the formula relative_power = (linear_power - linear_background)/ (linear_calibration - linear_background) as seen in the screen shot above.
• plotting the relative powers as a function of time, you get something similar to this:
• Change the guess values for the background and the calibrator powers until the empty sky signal appears at zero level in this plot, and the calibrator signal at 1.0.
• read off the value of the peak solar signal ... which is 4.8 in this example. This means that the solar signal is 4.8 times stronger than that of the calibrator, whose temperature will be about 290 K. Thus, we have determined the antenna temperature for the Sun as 4.8 * 290 K = 1390 K
• We need to emphasize here that this procedure is based on a simplified method of flux calibration, because we neglect completely the foreground of thermal emission in the Earth atmosphere. For the Sun this is quite premissible, because it is much brighter (1000 K) than the atmospheric noise (about 10 K)!

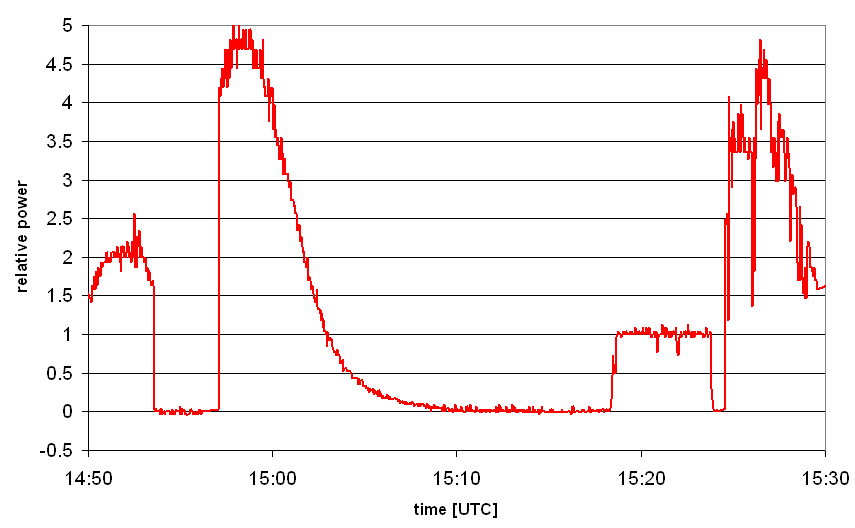
To complete the analysis, we need to determine the HPBW of our antenna. If you have been able to obtain a full drift scan, you could simply take the last plot and look up the times when the relative power was one half of the maximum value. If you did a half drift scan, and you are sure that you started exactly at the maximum signal, you could also look up the time that passed between the maximum and one half of the maximum value, then double this time. 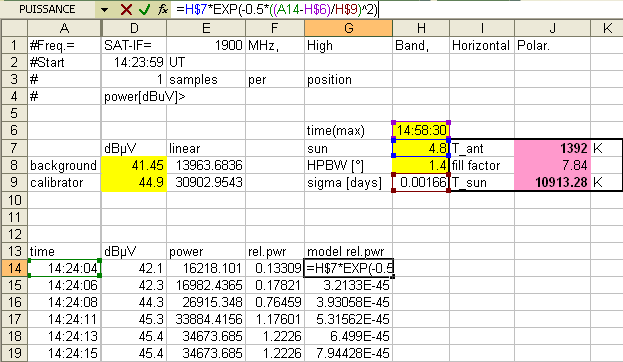But the neatest way is to match a simple model to your curve:

• We shall assume that the antenna pattern is described by a Gaussian function, so that the observed relative power behaves with time like this expression P(t) = linear_background + sunsignal*exp(-((t-tmax)/s)²/2) with sunsignal = T_ant(Sun)/290 K, the time tmax when the maximum occurred, and the width of the Gaussian s as free parameters
• If we measure s in units of days (as Excel treats times), the relation to the antenna's HPBW (measured in °) is s = HPBW/2.3548/360
• Put onto your worksheet a couple of more cells where you place these parameters, such as shown here:
• Add another column to contain relative powers predicted by the model, using the given formula, or as seen in the screenshot. Do this for all times of your data.
• Make another plot, with the observed and the model relative powers as function of time. You might add the model curve to your existing graph.
• Next, adjust the time of the maximum, the HPBW, and the guessed level of the solar signal until the model curve matches best your observations. With some cosmetics your final plot might look like this:
• With the antenna's HPBW known, we now can derive the temperature on the surface of the Sun: Since the Sun has an angular diameter of about 0.5°, much smaller than the antenna beam, its temperature must be correspondingly hotter than the Holiday Inn hotel which fills the entire antenna beam. Thus the true solar temperature is T_sun = T_ant(Sun) * (HPBW/0.5))² = 10900 K You may be surprised about such a high value ... but this is the temperature at the bottom of the Transition Layer, just above the Chromosphere, which is the layer of the Sun which we observe at 11 GHz!
• There are a few fine details: The Earth revolves once in 24 hours, or 15°/hr or 0.25°/min. However, the real speed across the sky of the Sun also depends on its actual declination d: it is somewhat slower speed of 0.25°/min*cos(d). In our example of data taken close to the spring equinox this correction is minute. Furthermore, the solar diameter also varies during the year, because the Earth's orbit is slightly elliptic. Declination and diameter of the Sun you may obtain from the here.
• Finally, we can derive the system temperature of the telescope, from the formula T_sys = T_cal / (linear_calibrator/linear_background - 1) = 290/(30900/13960 - 1) = 239 K This is a bit higher than we normally have for this instrument. The reason is that the background was higher due to the thermal emission of the gray rain clouds in the sky. On a sunny day, the background is as low as +40.7dBµV.

More about our results

Below we show one of our first drift scans with proper flux calibration. As described above, and in the Data Reduction, the raw data is corrected for the background and calibrated. The calibration measurement on the Holiday Inn hotel provides a temperature of about 300 K, hence the antenna temperature is 210 K.

To interpret our data, we need to understand how the measurements are related to the physical quantities (see Fundamental Concepts for the explanation of the technical terms):

What happens during calibration? Because the calibrator emits thermal radiation, the true temperature of the source is equal to the brightness temperature

Now let us consider what happens during the observation of the Sun: Again, the approximation of solar radiation by thermal emission of a certain temperature T_sun implies its equality with the brightness temperature

This determines our procedure:

• determine the half-power beam width of the antenna. Since the Sun moves with the sky in 4 time minutes by 1 deg, we measure the time that passes from the peak signal until the power has dropped to one half and multiply that angle by two. In the above example, we obtained a HPBW of about 3 deg.
• determine antenna temperature of the Sun, based on the calibration with the Holiday Inn measurement: 210 K
• correct for the ratio of the solid angles T_sun = T_ant_sun * W_ant / W_sun = T_ant_sun * (HPBW/0.5°)² and get the true temperature of the layers in the Sun that are responsable for the emission at 11 GHz: 210 * 36 = 7560 K.

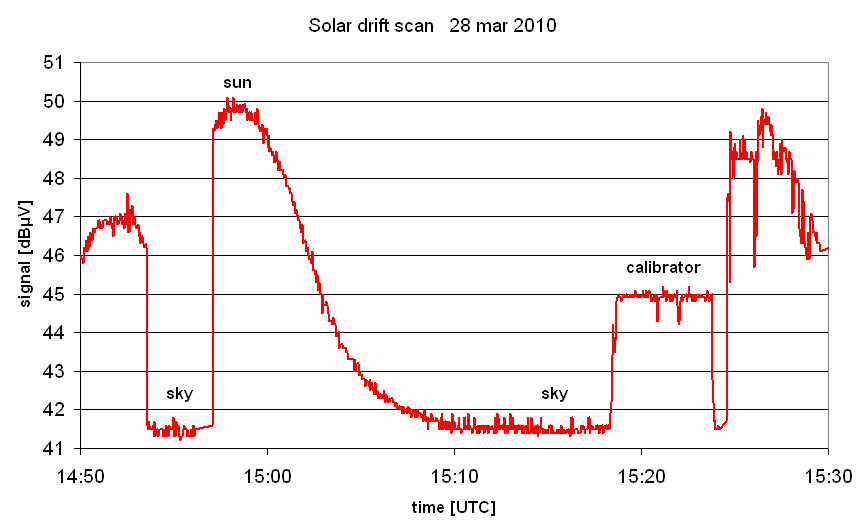
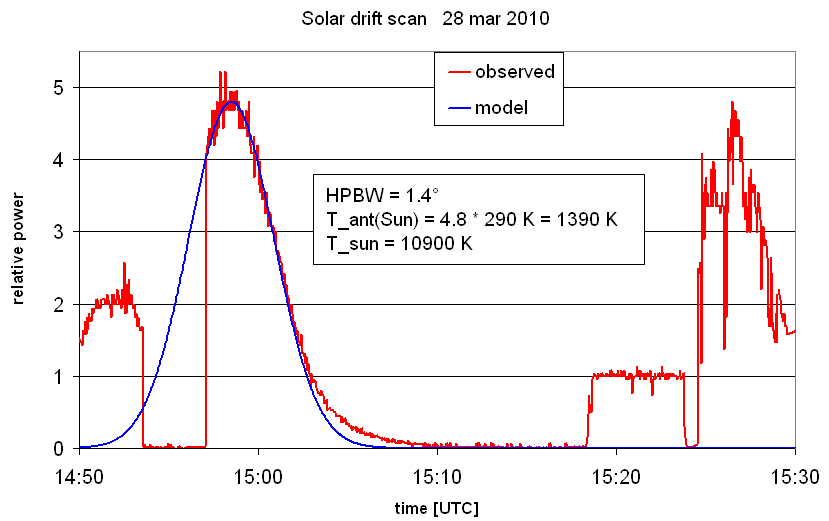
From the full drift scan shown on the right hand side of the above overview plot, we reduced the data which included taking into account the time changes of background and calibration levels, and we obtained the reduced data shown below:

The HPBW is determined as 3.75°, the solar antenna temperature of 210 K, and with a ratio of the solid angles of 56.3 the solar temperature is 11800 K. This is the temperature of the bottom of the corona, where the plasma is sufficiently transparent to let the 11 GHz radio waves escape. The value is in excellent agreement with literature data.

In the course of time, we became certain that our instrument did not perform as closely to the prototype as one could have expected. It turned out that the arm carrying the LNB had been bent upwards, as the telescope once got thrown over by strong wind gusts, and hence the dish was out of focus. Once the LNB arm had been straightened, the instrument performance came close to that of the prototype. For instance, the Sun has now a maximum signal of about 8 dB over the background level and the antenna beam is as narrow as expected. 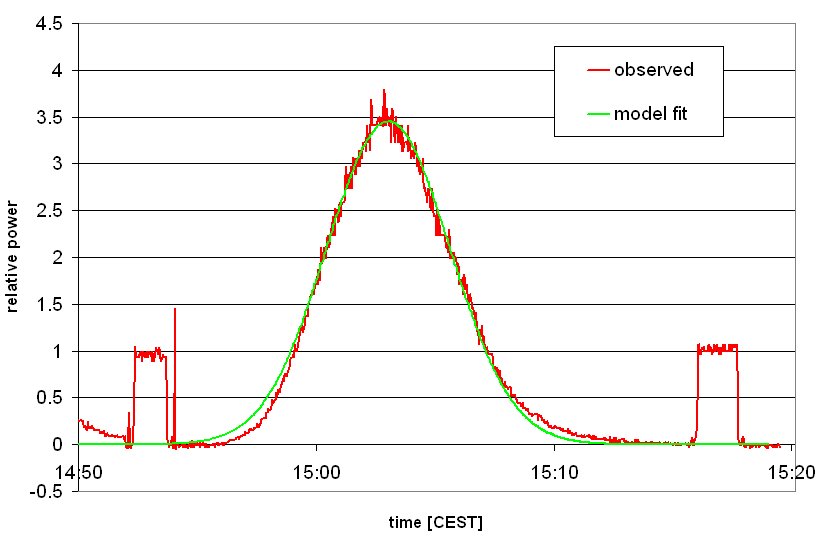A full drift scan of the Sun is shown here, along with the flux calibrations:

The HPBW now is only 1.5°, the solar antenna temperature 1035 K, and with a ratio of the solid angles of 9.3 the solar temperature is 9650 K. However, further observations did give higher temperatures, but also different values for the HPBW. Also, it is interesting to notice a small asymmetry of the antenna pattern ...

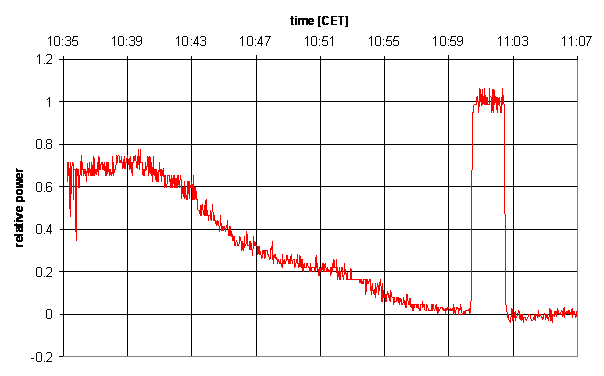
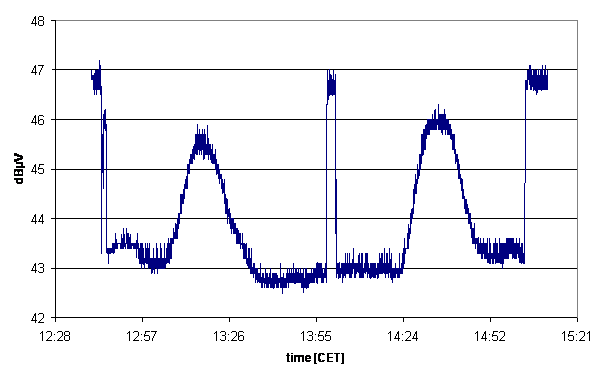
During our next observational campaign in 2008, Aikaterini Panopoulou conducted a careful study, measuring the peak solar signal many times, and under various weather conditions:

To capture the real peak may not be so easy: As explained above, we first search manually for the position with the highest signal. Because of the fluctuations of the signal, this requires some patience. Also, if we move the antenna a bit in one direction, then back in the other direction, this does not mean that we have returned exactly to the first position ... In the above figure we tried to capture the solar passage many times, after each passage searching again for the maximum signal and then going a bit to the right (and a bit downwards) to capture the sun again. As can be seen, while the absolute maximum is indeed just below +48.0 dBµV, several observations missed the sun by as much as 0.5 dB! An error of that size results in an error of 1600 K or about 15 percent in temperature!

| Top of the Page | Back to the MainPage | to my HomePage |

last update: May 2013 J.Köppen