Solar Flux, HPBW and System Temperature of ESA-Haystack (1.4 GHz)

Joachim Köppen, Strasbourg, 2011/12

Observations

Follow the procedures to switch-on the ESA-Haystack telescope

• Click Record to start recording the data
• Use any centre frequency, and a frequency span of 0.5 MHz
• Goto ISU for flux calibration and observe for 2 min.
• Goto the Sun, and observe for 2 min
• Offset the antenna from the Sun, by entering an offset angle into the field labelled PointCorr. Use either AZ or EL. Do this for offsets of e.g. -20, -16, …, 0, 4, 8, 12, and 20°, observe each position for 2 min
• Observe also the empty sky at the same elevation of the sun, but at azimuth at least 30° to the east

Interpretation (more details here and there):

• Import the text file with the data into Excel
• For each offset position do this
  • take the average spectrum of all the spectra recorded here, and plot it in a Scatter Plot
  • sum the powers over the spectrum – but do not use the first 9 and the last 9 frequency points
  • do the same for the empty sky, and determine its total power: Pcal.
  • The plot of the difference of these total powers and the total power taken at the empty sky, as a function of offset angle gives the shape of the main beam.
  • Fit a Gaussian curve to the data, and determine the width (note that HPBW = 2*sqrt(2*ln2) * σ)
• For the system temperature
  • Determine the total power for the calibrator: Pcal
  • Compute Tsys = 290 K / (Pcal / Psky – 1)
• Now that we have calibrated the instrument, we can also compute the flux of the sun
  • Compute the antenna temperature of the sun from
    Tant_sun = 290 K * (Pmax – Psky) / (Pcal – Psky)
  • The antenna beam’s solid angle is Ω = π/4 * (HPBW*π/180°)²
  • This gives its effective area: Aeff = λ²/Ω
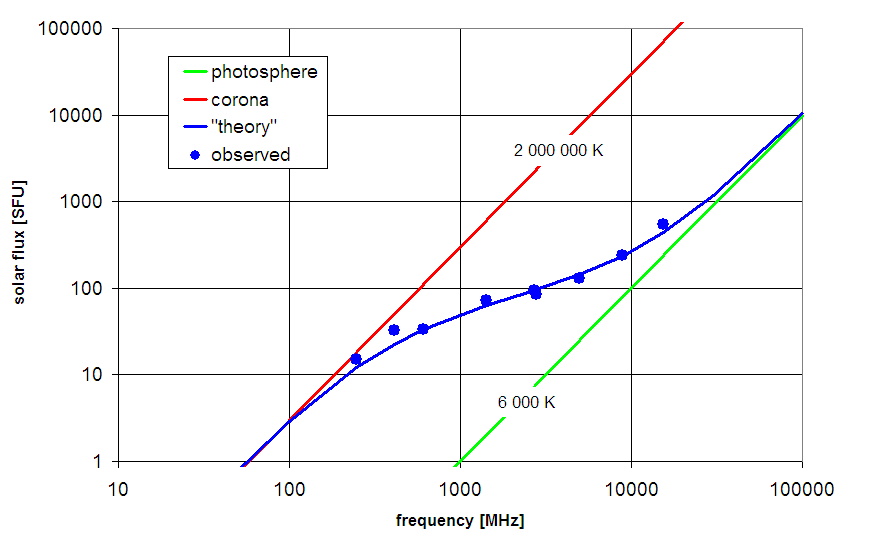
  • The flux F = 2 k Tant_sun/Aeff can be compared with the data for that day and frequency published by NOAA (note that 1 SolarFluxUnit = 10000 Jy)
• But it is very likely that our flux may differ from the NOAA flux. The reason is that our value for the effective area is not correct! We can use the flux measurements to compute the effective area of our telescope ... and then also compute its efficiency = Aeff/Ageometry. Values between 0.5 and 0.9 would be realistic.

• From here one gets the angular diameter of the sun: D.
• The filling factor with which the sun fills the antenna beam is given by: (D/HPBW)²
  which allows the compute the surface temperature from the maximum antenna temperature
  Tsun = Tant_sun * (D/HPBW)²

| back to Main page | ESA-Haystack Radio Telescope (1.4 GHz) | ESA-Dresden Ratio Telescope (10GHz) | to my HomePage |

last update: Sept. 2011 J.Köppen